Partial Differential Equation - Notes
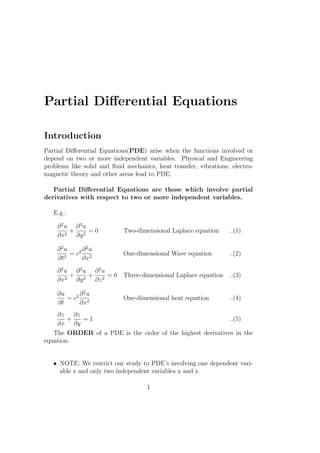
- 1. Partial Differential Equations Introduction Partial Differential Equations(PDE) arise when the functions involved or depend on two or more independent variables. Physical and Engineering problems like solid and fluid mechanics, heat transfer, vibrations, electro- magnetic theory and other areas lead to PDE. Partial Differential Equations are those which involve partial derivatives with respect to two or more independent variables. E.g.: ∂2 u ∂x2 + ∂2 u ∂y2 = 0 Two-dimensional Laplace equation ..(1) ∂2 u ∂t2 = c2 ∂2 u ∂x2 One-dimensional Wave equation ..(2) ∂2 u ∂x2 + ∂2 u ∂y2 + ∂2 u ∂z2 = 0 Three-dimensional Laplace equation ..(3) ∂u ∂t = c2 ∂2 u ∂x2 One-dimensional heat equation ..(4) ∂z ∂x + ∂z ∂y = 1 ..(5) The ORDER of a PDE is the order of the highest derivatives in the equation. • NOTE: We restrict our study to PDE’s involving one dependent vari- able z and only two independent variables x and y. 1
- 2. Partial Differential Equations • NOTATIONS: ∂z ∂x = p, ∂z ∂y = q, ∂2 z ∂x2 = r, ∂2 z ∂x∂y = s and ∂2 z ∂y2 = t Ex. 1 Show that u = sin9t sin(x 4 ) is a solution of a one dimensional wave equation. Sol. Here u = sin9t sin(x 4 ). ∂2 u ∂t2 = c2 ∂2 u ∂x2 .. (1) is one dimensional wave equation. ∂u ∂t = ∂2 u ∂t2 = ∂u ∂x = ∂2 u ∂x2 = Substitute above values in eq. (1), we get 2 Dept. of Mathematics, AITS - Rajkot
- 3. Partial Differential Equations c = ∴ For c = , u = sin9t sin(x 4 ) is a solution of a wave equation. Ex. 2 Verify that the function u = ex cosy is the solution of the Laplace equation ∂2 u ∂x2 + ∂2 u ∂y2 = 0. Sol. Dept. of Mathematics, AITS - Rajkot 3
- 4. Partial Differential Equations Ex. 3 Verify that the function u = x3 + 3xt2 is the solution of the wave equation ∂2 u ∂t2 = c2 ∂2 u ∂x2 for a suitable value of c. Sol. 4 Dept. of Mathematics, AITS - Rajkot
- 5. Partial Differential Equations Exercise 1.1 Q.1 Verify the following functions are the solutions of the Laplace’s equation ∂2 u ∂x2 + ∂2 u ∂y2 = 0. (a) u = log(x2 + y2 ) (b) u = sinxsinhy (c) u = tan−1 y x Q.2 Verify the following functions are the solutions of the wave equation ∂2 u ∂t2 = c2 ∂2 u ∂x2 for a suitable value of c. (a) u = x3 + 3xt2 (b) u = cosctsinx Q.3 Verify the following functions are the solutions of the heat equation ∂u ∂t = c2 ∂2 u ∂x2 for a suitable value of c. (a) u = e−2t cosx Dept. of Mathematics, AITS - Rajkot 5
- 6. Partial Differential Equations Formation of PDE Partial Differential equations can be formed either by the elimination of (1) arbitrary constants present in the functional relation present in the rela- tion between the variable (2) arbitrary functions of these variables. By elimination of arbitrary constants Consider the function f(x, y, z, a, b) = 0 ..(i) where a and b are two independent arbitrary constants. To eliminate two constants, we require two more equations. Differentiating eq. (i) partially with respect to x and y in turn, we obtain ∂f ∂x + ∂f ∂z ∂z ∂x = 0 ⇒ ∂f ∂x + p ∂f ∂z = 0 .. (ii) ∂f ∂y + ∂f ∂z ∂z ∂y = 0 ⇒ ∂f ∂y + q ∂f ∂z = 0 .. (iii) Eliminating a and b from the set of equations (i), (ii) and (iii) , we get a PDE of the first order of the form F(x, y, z, p, q) = 0. .. (iv) Ex. 1 Eliminate the constants a and b form z = (x + a)(y + b). Sol. Given that z = (x + a)(y + b) ... (1) Differentiating partially eq. (1) with respect to x and y respectively, we get ∂z ∂x = p = ..(2) ∂z ∂y = q = ..(3) Eliminating a and b from equation (1), (2) and (3), we get 6 Dept. of Mathematics, AITS - Rajkot
- 7. Partial Differential Equations is the required partial differential equation. Ex. 2 Find the differential equation of the set of all spheres whose centres lie on the z-axis. Sol. The equation of the spheres with centres on the z-axis is ..(1) Differentiating partially eq. (1) with respect to x and y respectively, we get Eliminating arbitrary constant from (2) and (3), we get is the required partial differential equation. Dept. of Mathematics, AITS - Rajkot 7
- 8. Partial Differential Equations By elimination of arbitrary functions Consider a relation between x, y and z of the type φ(u, v) = 0 ..(v) where u and v are known functions of x, y and z and φ is an arbitrary function of u and v. Differentiating equ. (v) partially with respect to x and y, respectively, we get ∂φ ∂u ∂u ∂x + ∂u ∂z p + ∂φ ∂v ∂v ∂x + ∂v ∂z p = 0 .. (vi) ∂φ ∂u ∂u ∂y + ∂u ∂z q + ∂φ ∂v ∂v ∂y + ∂v ∂z q = 0 .. (vii) Eliminating ∂φ ∂u and ∂φ ∂v from the eq. (vi) and (vii), we get the equations ∂(u, v) ∂(y, z) p + ∂(u, v) ∂(z, x) q = ∂(u, v) ∂(x, y) .. (viii) which is a PDE of the type (iv). since the power of p and q are both unity it is also linear equation, whereas eq. (iv) need not be linear. Ex. 1 Eliminate the arbitrary function from the equation z = xy + f(x2 + y2 ). Sol. Let x2 + y2 = u. ∴ z = xy + f(u) .. (1) differentiating eq. (1) with respect to x and y respectively, we get 8 Dept. of Mathematics, AITS - Rajkot
- 9. Partial Differential Equations p = ..(2) q = ..(3) eliminating from (2) and (3), we get Ex. 2 Eliminate arbitrary function from the equation f(x + y + z, x2 + y2 + z2 ) = 0. Sol. Let x + y + z = u, x2 + y2 + z2 = v then f(u, v) = 0 .. (1) Differentiating (1) with respect to x and y, we get ∂f ∂u ∂u ∂x + ∂u ∂z p + ∂f ∂v ∂v ∂x + ∂v ∂z p = 0 .. (2) ∂f ∂u ∂u ∂y + ∂u ∂z q + ∂f ∂v ∂v ∂y + ∂v ∂z q = 0 .. (3) Now ∂u ∂x = ∂v ∂x = ∂u ∂y = ∂v ∂y = ∂u ∂z = ∂v ∂z = Dept. of Mathematics, AITS - Rajkot 9
- 10. Partial Differential Equations substituting above values in eq. (2) and (3), we get eliminating and from above eq. (4) and (5), we get is the required partial differential equation of the first order. 10 Dept. of Mathematics, AITS - Rajkot
- 11. Partial Differential Equations Exercise 1.2 Q.1 Form the partial differential equation by eliminating the arbitrary constants from the following: 1. z = (x2 + a)(y2 + b) 2. (x − a)2 + (y − b)2 + z2 = 1 Q.2 Form the partial differential equations by eliminating the arbitrary functions from the following: 1. z = f(x2 − y2 ) 2. z = x + y + f(xy) 3. f(xy + z2 , x + y + z) = 0 4. f(x2 + y2 + z2 , xyz) = 0 Dept. of Mathematics, AITS - Rajkot 11
- 12. Partial Differential Equations Integrals of Partial Differential Equation • A solution or integral of a partial differential equation is a relation between the dependent and the independent variables that satisfies the differential equation. • A solution which contains a number of arbitrary constants equal to the independent variables, is called a complete integral. • A solution obtained by giving particular values to the arbitrary constants in a complete integral is called a particular integral. • Singular integral Let F(x, y, z, p, q) = 0 .. (1) be the partial differential equation whose complete integral is f(x, y, z, a, b) = 0 .. (2) eliminating a, b between eq. (2) and ∂f ∂a = 0, ∂f ∂b = 0 if it exists, is called a singular integral. • General Integral In eq. (2), if we assume b = φ(a), then (2) becomes f[x, y, z, a, φ(a)] = 0 .. (3) differentiating (2) partially with respect to a, ∂f ∂a + ∂f ∂b φ (a) = 0 .. (4) eliminating a between theses two equations (3) and (4), if it exists, is called the general integral of eq. (1). 12 Dept. of Mathematics, AITS - Rajkot
- 13. Partial Differential Equations Solutions of PDE by the method of Direct Integration This method is applicable to those problems, where direct integration is possible. Ex. 1 Solve ∂2 z ∂x∂y = x3 + y3 Sol. Given that ∂ ∂x ∂z ∂y = x3 + y3 Integrating w.r.t to keeping constant, we get ∴ ∂z ∂y = Now integrating w.r.t. to keeping as constant ∴ z = Dept. of Mathematics, AITS - Rajkot 13
- 14. Partial Differential Equations Ex. 2 Solve ∂2 u ∂x∂t = e−t cosx Sol. Given that ∂ ∂x ∂u ∂t = e−t cosx Integrating w.r.t to keeping constant, we get Now integrating w.r.t. to keeping as constant 14 Dept. of Mathematics, AITS - Rajkot
- 15. Partial Differential Equations Ex. 3 Solve ∂3 z ∂x2∂y = cos(2x + 3y) Sol. Given that ∂2 ∂x2 ∂z ∂y = cos(2x + 3y) Integrating w.r.t to keeping constant, we get ∴ ∂ ∂x ∂z ∂y = Integrating w.r.t. to keeping as constant Finally integrating w.r.t. to keeping as constant Dept. of Mathematics, AITS - Rajkot 15
- 16. Partial Differential Equations Lagrange’s Equation We have ∂(u, v) ∂(y, z) p + ∂(u, v) ∂(z, x) q = ∂(u, v) ∂(x, y) This can be expressed in the form Pp + Qq = R, where P, Q and R are functions of x, y and z. This partial differential equation is known as Lagrange’s equation. Method to solve Pp + Qq = R In order to solve the equation Pp + Qq = R 1 Form the subsidiary (auxiliary ) equation dx P = dy Q = dz R 2 Solve these subsidiary equations by the method of grouping or by the method of multiples or both to get two independent solutions u = c1 and v = c2. 3 Then φ(u, v) = 0 or u = f(v) or v = f(u) is the general solution of the equation Pp + Qq = R. Ex. 1 Solve y2 z x ∂z ∂x + xz ∂z ∂y = y2 . Sol. The given equation can be written as ( in for of p and q) y2 zp + x2 zq = xy2 comparing with Pp + Qq = R, we get P = , Q = and R = The subsidiary equations are dx P = dy Q = dz R Taking first two, we have 16 Dept. of Mathematics, AITS - Rajkot
- 17. Partial Differential Equations Now taking first and third, we have Ex. 2 Find the general solution of the differential equation x2 p + y2 q = (x + y)z Sol. Comparing with Pp + Qq = R, we get P = , Q = and R = The subsidiary equations are dx P = dy Q = dz R Dept. of Mathematics, AITS - Rajkot 17
- 18. Partial Differential Equations Taking first two, we have Ex. 3 Solve (y + z)p + (z + x)q = x + y Ex. 4 Solve pz − qz = z2 + (x + y)2 Ex. 5 Solve x2 (y − z)p + y2 (z − x) = z2 (x − y) Ex. 6 Solve (z2 − 2yz − y2 )p + (xy + zx)q = xy − zx Exercise 1. xp + yq = z 2. xp − yq = xy 3. (1 − x)p + (2 − y)q = 3 − z 4. zxp − zyq = y2 − x2 5. (y − z)p + (z − x)q = x − y 6. p − 2q = 2x − ey + 1 7. x(y2 − z2 )p + y(z2 − x2 )q = z(x2 − y2 ) 18 Dept. of Mathematics, AITS - Rajkot
- 19. Partial Differential Equations Special type of Nonlinear PDE of the first order A PDE which involves first order derivatives p and q with degree more than one and the products of p and q is called a non-linear PDE of the first order. There are four standard forms of these equations. 1. Equations involving only p and q 2. Equations not involving the independent variables 3. Separable equations 4. Clairaut’s form Standard Form 1. Equations involving only p and q Such equations are of the form f(p,q)=0 z = ax + by + c ... (1) is a solution of the equation f(p, q) = 0 provided f(a, b) = 0 (means put p = a and q = b) ... (2) solving (2) for b, b = F(a) Hence the complete integral is z = ax + F(a)y + c where a and c are arbitrary constants. Ex. 1 Solve pq = p + q Sol. Here the give DE involves on p and q (so standard form 1) The solution is put p = and q = therefore, b = Dept. of Mathematics, AITS - Rajkot 19
- 20. Partial Differential Equations Hence the complete solution is Exercise Solve the following equations 1. pq = 1 2. p = q2 3. p2 + q2 = 4 4. pq + p + q = 0 20 Dept. of Mathematics, AITS - Rajkot
- 21. Partial Differential Equations Standard Form 2. Equations not involving the independent variables Such equations are of the form f(z,p,q)=0 Consider f(z, p, q) = 0 .. (1) since z is a function of x and y dz = ∂z ∂x dx + ∂z ∂y dy = pdx + qdy let q = ap then eq (1) becomes f(z, p, ap) = 0 .. (2) solving (2) for p i.e. p = φ(z, a) ∴ dz = φ(z, a) dx + a φ(z, a) dy ∴ dz φ(z, a) = dx + ady integrating, we get dz φ(z, a) = x + ay + b which is a complete integral. Ex. 1 Solve: p2 z2 + q2 = 1 Sol. Given equation involves only p, q and z (standard form 2) Therefore putting q = ap in given equation, we get Now dz = pdx + qdy, substituting above values in this equation and integrate Dept. of Mathematics, AITS - Rajkot 21
- 22. Partial Differential Equations Ex. 2 Solve: p(1 + q) = qz Ex. 3 Solve: z = p2 + q2 Exercise 1 q(p2 z + q2 ) = 4 2 pq = z2 3 p + q = z 4 zpq = p + q 5 z2 = 1 + p2 + q2 22 Dept. of Mathematics, AITS - Rajkot
- 23. Partial Differential Equations Standard Form 3. Separable equations Such equations are of the form f1(x,p) = f2(y,q) A first order PDE is seperable if it can be written in the form f1(x, p) = f2(y, q) We assume each side equal to an arbitrary constant a. solving f1(x, p) = a ⇒ p = φ1(x, a) solving f2(y, q) = a ⇒ q = φ2(y, a) ∴ z = φ1(x, a)dx + φ2(y, a)dy which is complete integral Ex. 1 Solve: p − x2 = q + y2 Sol. Writing equation in the form p − x2 = q + y2 = a ∴ p = a + x2 and q = a − y2 Now dz = pdx + qdy dz = dx + dy Integrating both sides, we get z = Ex. 2 Solve: p2 + q2 = x2 + y2 Sol. Writing equation in the form p2 − x2 = y2 − q2 Let p2 − x2 = a and y2 − q2 = a Hence, p2 = x2 + a, q2 = y2 − a Dept. of Mathematics, AITS - Rajkot 23
- 24. Partial Differential Equations ∴ p = and q = Now dz = pdx + qdy dz = Exercise 1. q − p + x − y = 0 2. p + q = x + y 3. P2 + q2 = x + y 4. pq = xy 5. py + qx = pq 6. p + q = sinx + siny 24 Dept. of Mathematics, AITS - Rajkot
- 25. Partial Differential Equations Standard Form 4. Clairaut’s form A first-order PDE is said to be of Clairaut type if it can be written in the form, z = px + qy + f(p, q) substitute p = a and q = b in f(p, q) The solution of the the equation is z = ax + by + f(a, b) Ex. 1 Solve: z = px + qy + 1 + p2 + q2 Sol. The complete integral is obtained z = px + qy + √ 1 + a2 + b2 Ex. 2 Solve: (p + q)(z − xp − yq) = 1. Sol. Exercise Solve the following equations. 1. z = px + qy + p2 q2 2. z = px + qy + 2 √ pq 3. z = px + qy + q p − p 4. (1 − x)p + (2 − y)q = 3 − z 5. z pq = x q + y p + √ pq Dept. of Mathematics, AITS - Rajkot 25
- 26. Partial Differential Equations Classification of the PDE of second order Consider the equation of the second order as A ∂2 u ∂x2 + B ∂2 u ∂x∂y + C ∂2 u ∂2y + f x, y, u, ∂u ∂x , ∂u ∂y = 0 (1) where A is positive Now the equation (1) is (i) elliptic if B2 − 4AC < 0 (ii) hyperbolic if B2 − 4AC > 0 (iii) parabolic if B2 − 4AC = 0. Exercise Classify the following differential equations. 1 2 ∂2 u ∂t2 + 4 ∂2 u ∂x∂t + 3 ∂2 u ∂x2 = 0 2 4 ∂2 u ∂t2 − 9 ∂2 u ∂x∂t + 5 ∂2 u ∂x2 = 0 3 ∂2 u ∂t2 + 4 ∂2 u ∂x∂t + 4 ∂2 u ∂x2 = 0 26 Dept. of Mathematics, AITS - Rajkot
