Algebraic Fractions and Polynomial Factorization
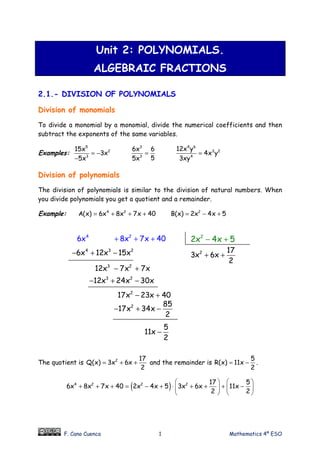
- 1. F. Cano Cuenca 1 Mathematics 4º ESO Unit 2: POLYNOMIALS. ALGEBRAIC FRACTIONS 2.1.- DIVISION OF POLYNOMIALS Division of monomials To divide a monomial by a monomial, divide the numerical coefficients and then subtract the exponents of the same variables. Examples: 5 2 3 15x 3x 5x = − − 3 3 6x 6 55x = 4 6 3 2 4 12x y 4x y 3xy = Division of polynomials The division of polynomials is similar to the division of natural numbers. When you divide polynomials you get a quotient and a remainder. Example: 4 2 A(x) 6x 8x 7x 40= + + + 2 B(x) 2x 4x 5= − + The quotient is 2 17 Q(x) 3x 6x 2 = + + and the remainder is 5 R(x) 11x 2 = − . ( )4 2 2 2 17 5 6x 8x 7x 40 2x 4x 5 3x 6x 11x 2 2 + + + = − + ⋅ + + + − 4 2 6x 8x 7x 40+ + + 2 2x 4x 5− + 4 3 2 6x 12x 15x− + − 2 17 3x 6x 2 + + 3 2 12x 24x 30x− + − 2 85 17x 34x 2 − + − 5 11x 2 − 3 2 12x 7x 7x− + 2 17x 23x 40− +
- 2. F. Cano Cuenca 2 Mathematics 4º ESO In general, if you divide the polynomial A(x) by the polynomial B(x) and the quotient and the remainder are Q(x) and R(x) respectively, you can write that: A(x) B(x) Q(x) R(x)= ⋅ + . When the remainder is 0, we have that A(x) B(x) Q(x)= ⋅ . In this case, the polynomial A(x) is divisible by B(x), that is, B(x) is a factor or divisor of A(x). Exercise 1: Work out the following divisions of polynomials. a) ( ) ( )5 4 3 2 x 7x x 8 : x 3x 1− + − − + b) ( ) ( )5 4 3 2 2 4x 20x 18x 28x 28x 6 : x 5x 3+ − − + − + − c) ( ) ( )4 2 2 6x 3x 2x : 3x 2+ − + d) ( ) ( )2 3 2 45x 120x 80x : 3x 4+ + + Division of a polynomial by x-a. Ruffini’s rule It is very common to divide a polynomial by x a− . For example: The quotient is 3 2 7x 10x 30x 4+ + − and the remainder is 5− . ( )( ) ( )4 3 3 2 7x 11x 94x 7 x 3 7x 10x 30x 4 5− − + = − + + − + − A(x) B(x) Q(x)R(x) − − +4 3 7x 11x 94x 7 x 3− − +4 3 7x 21x + + −3 2 7x 10x 30x 4 − +3 2 10x 30x − +2 30x 90x − +4x 7 3 10x −2 30x 94x −4x 12 −5
- 3. F. Cano Cuenca 3 Mathematics 4º ESO But this division can also be done using Ruffini’s rule: We start writing the coefficients of the dividend and the number a. QUOTIENT: 7 10 30 4− , that is, 3 2 7x 10x 30x 4+ + − REMAINDER: 5− Notice that Ruffini’s rule’s steps are exactly the same as the steps of the long division. The advantage of Ruffini’s rule is that you only work with the coefficients and only do the essential operations. IMPORTANT!! Exercise 2: Use Ruffini’s rule for doing the following divisions of polynomials. a) ( ) ( )4 2 5x 6x 11x 13 : x 2+ − + − b) ( ) ( )5 4 6x 3x 2x : x 1− + + c) ( ) ( )4 3 2 3x 5x 7x 2x 13 : x 4− + − + − d) ( ) ( )4 3 2 6x 4x 51x 3x 9 : x 3+ − − − + QUOTIENT ’S COEFFICIENTS REMAINDER Ruffini’s rule only works when you divide a polynomial by a linear factor x a− .
- 4. F. Cano Cuenca 4 Mathematics 4º ESO 2.2.- RUFFINI’S RULE’S USES Look at the division ( ) ( )3 2 2x 8x 31x 42 : x 6− − + − 2 -8 -31 42 6 12 24 ( 7) 6− ⋅ 2 4 -7 0 The quotient is 2 2x 4x 7+ − and the remainder is 0. Therefore, you can write that ( )( )3 2 2 2x 8x 31x 42 x 6 2x 4x 7− − + = − + − . Then, ( )x 6− is a factor of the polynomial 3 2 2x 8x 31x 42− − + , that is, the polynomial 3 2 2x 8x 31x 42− − + is divisible by ( )x 6− . Notice that 6 is a divisor of 42. So if you are looking for factors of a polynomial P(x), have a try with the linear factors (x a)− where “a” is a divisor of the constant term of P(x). Exercise 3: Find two linear factors of the polynomial 4 3 2 x 3x 2x 10x 12+ − − − . Exercise 4: Check if the following polynomials are divisible by x 3− or x 1+ . a) 3 2 A(x) x 3x x 3= − + − b) 3 2 B(x) x 4x 11x 30= + − − c) 4 3 2 C(x) x 7x 5x 13= − + − The Remainder Theorem Remember that you can calculate the number value of a polynomial at a given value of the variable. When the coefficients of a polynomial P(x) are integers, if(x a)− is a factor of P(x) and “a” is also an integer number, then “a” is a divisor of the constant term of P(x).
- 5. F. Cano Cuenca 5 Mathematics 4º ESO Example: Calculate the number value of 3 2 P(x) 2x x 4x 2= − − + at x 3= − ( ) ( ) ( ) ( ) 3 2 P( ) 2 4 2 2 27 9 123 3 3 2 54 9 12 2 493= − − + = ⋅ − − + + = − − + +− − = −− − The Remainder Theorem states: Proof: If x a P(a) (a a) Q(a) R R= ⇒ = − ⋅ + = P(a) R⇒ = Exercise 5: Use Ruffini’s rule to calculate P(a) in the following cases. a) 4 2 P(x) 7x 5x 2x 24= − + − , a 5= − , a 10= b) 3 2 P(x) 3x 8x 3x= − + , a 1= , a 8= Exercise 6: Find the value of m so that the polynomial 3 2 P(x) x mx 5x 2= − + − is divisible by x 1+ . Exercise 7: The remainder of the division ( ) ( )4 3 2x kx 7x 6 : x 2+ − + − is 8− . What is the value of k? 2.3.- FACTORIZING POLYNOMIALS Roots of a polynomial A number “a” is called a root of a polynomial P(x) if P(a) 0= . The roots (or zeroes) of a polynomial are the solutions of the equation P(x) 0= . Examples: a) The numbers 1 and 1− are roots of the polynomial 2 P(x) x 1= − . 2 P(1) 1 1 0= − = 2 P( 1) ( 1) 1 0− = − − = b) Find the roots of the polynomial 2 P(x) x 5x 6= − + . The number value of the polynomial P(x) at x a= is the same as the remainder of the division P(x) : (x a)− . That is, P(a) R= . P(x) (x a) Q(x) R= − ⋅ +
- 6. F. Cano Cuenca 6 Mathematics 4º ESO The roots of P(x) are the solutions of the equation P(x) 0= . 2 1 2 x 2 P(x) 0 x 5x 6 0 x 3 = = ⇒ − + = ⇒ = The roots of the polynomial 2 P(x) x 5x 6= − + are 2 and 3. One of the most important uses of Ruffini’s rule is to find the roots of a polynomial. Remember that the remainder of the division P(x) : (x a)− is the same as P(a). Therefore, if the remainder is 0, then P(a) 0= , so the number “a” is a root of P(x). Examples: Find a root of the polynomial 4 3 2 P(x) x 2x 7x 8x 12= + − − + 1 2 -7 -8 12 1 1 3 -4 -12 1 3 -4 -12 0 The remainder of the division P(x) : (x 1)− is 0. Then, 1 is a root of P(x). An interesting theorem about polynomials is the Fundamental Theorem of Algebra: Let n n 1 n 2 2 n n 1 n 2 2 1 0 P(x) a x a x a x ... a x a x a− − − − = + + + + + + be any polynomial of degree n. If 1 2 3 n r, r , r ,...,r are its n roots, then the polynomial can be factorized as: Example: Find the roots of the polynomial 2 P(x) 2x x 1= − − and factorize it. 1 2 2 x 1 P(x) 0 2x x 1 0 1 x 2 = = ⇒ − − = ⇒ = − If the remainder of the division P(x) : (x a)− is 0, then the number “a” is a root of P(x). Any polynomial of degree n has n roots. (These roots can be real or complex numbers). n 1 2 n 1 n P(x) a (x r) (x r ) .... (x r ) (x r )− = − ⋅ − ⋅ ⋅ − ⋅ −
- 7. F. Cano Cuenca 7 Mathematics 4º ESO The roots of 2 P(x) 2x x 1= − − are 1 and 1 2 − . The factorization of P(x) is ( ) ( )1 1 P(x) 2 x 1 x 2 x 1 x 2 2 = − − − = − + . Factorization techniques You have seen before the factorization of a polynomial in the previous example. Factorize a polynomial means to write it as a product of lower degree polynomials. Examples: ( )( )2 x 9 x 3 x 3− = + − ( )( )3 2 x 5x 6x x x 2 x 3− + = − − There are different techniques for factorizing polynomials: 1) Taking out common factor. Example: ( )2 8x 2x 2x 4x 1− = − 2) Using polynomial identities (the square of the sum, the square of the difference, the product of a sum and a difference). Examples: ( ) ( )( ) 22 x 10x 25 x 5 x 5 x 5+ + = + = + + ( ) ( )( ) 22 x 6x 9 x 3 x 3 x 3− + = − = − − ( )( )2 x 16 x 4 x 4− = + − 3) Using the Fundamental Theorem of Algebra. Example: The roots of the polynomial 2 P(x) x x 6= − − + are 2 and 3− . ( )( )( )2 P(x) x x 6 1 x 2 x 3= − − + = − − + Exercise 8: Take out common factor or use the polynomial identities to factorize the following polynomials. a) 2 3x 12x− b) 3 2 4x 24x 36x− + c) 2 4 45x 5x− d) 4 2 3 x x 2x+ + e) 6 2 x 16x− f) 4 16x 9− Exercise 9: Use the Fundamental Theorem of Algebra to factorize the following polynomials. a) 2 x 4x 5+ − b) 2 x 8x 15+ + c) 2 7x 21x 280− − d) 2 3x 9x 210+ −
- 8. F. Cano Cuenca 8 Mathematics 4º ESO 4) Using Ruffini’s rule. Example: 3 2 P(x) x 2x 5x 6= − − + ( )( ) ( )( )( )3 2 2 P(x) x 2x 5x 6 x 1 x x 6 x 1 x 2 x 3= − − + = − − − = − + − 5) A combination of the previous ones. Examples: a) ( ) ( ) 25 4 3 3 2 3 P(x) 12x 36x 27x 3x 4x 12x 9 3x 2x 3= − + = − + = − If we solve the equation P(x) 0= , we get the roots of P(x). ( ) ( ) ( ) 23 P(x) 0 3x 2x 3 0 3 x x x 2x 3 2x 3 0= ⇒ − = ⇒ ⋅ ⋅ ⋅ ⋅ − ⋅ − = ⇒ 1 2 3 4 5 3 3 x 0, x 0, x 0, x , x 2 2 ⇒ = = = = = The polynomial has a double root and a triple root. b) 3 P(x) x x 6= − − Firstly, we use Ruffini’s rule: Secondly, we find the roots of the polynomial 2 x 2x 3− + . 1 -2 -5 6 1 1 -1 -6 1 -1 -6 0 -2 -2 6 1 -3 0 Taking out common factor Using polynomial identities 1 0 -1 6 -2 -2 4 -6 1 -2 3 0
- 9. F. Cano Cuenca 9 Mathematics 4º ESO 2 x 2x 3 0− + = a 1, b 2, c 3= = − = ( ) 2 2 2 4 1 3 2 8 x 2 1 2 ± − − ⋅ ⋅ ± − = = ⋅ The roots of the polynomial 2 x 2x 3− + are not real numbers. So the factorization of P(x) is ( )( )2 P(x) x 2 x 2x 3= + − + . Exercise 10: Factorize the following polynomials. a) 2 3x 2x 8+ − b) 5 3x 48x− c) 3 2 2x x 5x 12+ − + d) 3 2 x 7x 8x 16− + + e) 4 3 2 x 2x 23x 60x+ − − f) 4 3 2 9x 36x 26x 4x 3− + + − Exercise 11: Take out common factor in the following expressions. a) 3x(x 3) (x 1)(x 3)− − + − b) (x 5)(2x 1) (x 5)(2x 1)+ − + − − c) (3 y)(a b) (a b)(3 y)− + − − − Exercise 12: Write second degree polynomials whose roots are: a) 7 and -7 b) 0 and 5 c) -2 and -3 d) 4 (double) Exercise 13: Prove that the polynomial n x 1− is divisible by x 1− for any value of n. Find the general expression for the quotient of this division. Exercise 14: The remainder of the division 2 P(x) : (x 1)− is 4x 4+ . Find the remainder of the division P(x) : (x 1)− . Exercise 15: Prove that the polynomial 2 x (a b)x ab+ + + is divisible by x a+ and by x b+ for any values of a and b. Find its factorization. 2 8i 2 2 2i2 8 1 2i 2 2 2 + ++ − = = = + ∉ » 2 8i 2 2 2i2 8 1 2i 2 2 2 − −− − = = = − ∉ »
- 10. F. Cano Cuenca 10 Mathematics 4º ESO 2.4.- ALGEBRAIC FRACTIONS An algebraic fraction is the quotient of two polynomials, that is, P(x) Q(x) Examples: 2 2x 4 x 5x 3 + − + 1 x 5− 4 3 2 x 5x 1 x x − + + 2x 5 11 + The same calculations that you do with numerical fractions can be done with algebraic fractions. Simplification of algebraic fractions To simplify algebraic fractions: • Factorize the polynomials. • Cancel out the common factors. Example: ( )2 2 x 1x 1 x 2x 3 +− = − − ( ) ( ) x 1 x 1 − + ( ) x 1 x 3x 3 − = −− Addition, subtraction, product and division of algebraic fractions You can add, subtract, multiply an divide algebraic fractions in the same way that you do in simple arithmetic. Examples: a) ( ) ( )( ) ( ) ( ) ( ) ( ) 2 22 x 7 x 2 2x 1 x x 1 x 1 x 7 x 2 x 2x 1 2x 7x 9 x x 1x 1 x x 1x + + −+ − − − + − + + + + = + = + + + b) ( )( ) ( ) 2 2 3 2 3 222 x 2 x 1 xx x 2x 2 x x 2 2x x 2 2 x 1 2x 1 xx 2 1x xx + − − + −+ − + − = = = + ++ ⋅ + c) ( )( ) ( )( ) ( ) ( ) 2 2 x 5 x x 2x 5 x 2x x x 5 2x 1 : x 2 x 2x 12x 2 + ++ + = = ++ + + − + ( )x 2+ ( ) ( ) 2x 5 x x 5x 2x 1 2x 12x 1 + + = = + ++ d) ( )( ) ( ) ( )( )( ) ( ) ( ) 2 2 2 2 2 2 x 9 xx 9 x 4 x 9 x 4 x 2x 3 : x 2x 2 4 x 3 : x x x2 x x 2 x2 x 3 − − − − −− = = = −+− + − − ⋅ − − + ( ) ( )x 3 x 3+ − = ( )x 2+ ( )( ) ( ) x 2 x 2 x 2 − − + ( )x x 3− 3 2 x x 8x 12 x − − + = ( )LCM x, x(x 1), (x 1) x(x 1)− − = −
- 11. F. Cano Cuenca 11 Mathematics 4º ESO Exercise 16: Simplify the following algebraic fractions. a) 2 3 2x 6x 4x 2x − − b) ( ) ( ) ( ) ( ) 2 2 x 3 x x 3 x 3 x x 2 − + − + c) 3 2 3 2 x 3x x 3 x 3x + + + + d) 3 2 3 2 x 5x 6x x x 14x 24 − + − − + Exercise 17: Work out and simplify. a) 2 2 2x 1 x 5 x 3 x 3x + + − + + b) 2 2 3 x x x x 1 x 1 − + − c) 2 5x 10 x 9 x 3 x 2 − − ⋅ + − d) 2 3x 1 x 3 2x 5 x x 2x 2x − + + − + −− e) 2 2x 1 x : 2x 1 4x 2 + − − f) 2 x 1 1 : x 1 x x 1 − − − Exercise 18: Translate into algebraic language. (You can only use one unknown). a) The quotient between two even consecutive numbers. b) A number minus its inverse. c) The inverse of a number plus the inverse of twice that number. d) The sum of the inverses of two consecutive numbers. Exercise 19: Use polynomials to express the area and the volume of this prism. Exercise 20: A tap takes x minutes to fill a tank. Another tap takes 3 minutes less than the first one to fill the same tank. Express as a function of x the part of the tank to be filled if we turn both taps on for one minute.
- 12. F. Cano Cuenca 12 Mathematics 4º ESO Exercise 21: We mix “x” kg of a paint that costs 5 €/kg with “y” kg of another paint that costs 3 €/k. Express the price of 1 kg of the mixture as a function of “x” and “y”. Exercise 22: Two cyclists start at the same time from opposite ends of a course that is 60 miles long. One cyclist is riding at x mph and the second cyclist is riding at x 3+ mph. How long after they begin will they meet? (Give the answer as a function of x). Exercise 23: A rhombus is inscribed in a rectangle whose sides are x an y. Express the perimeter of the rhombus as a function of x and y. Exercise 24: The sides AB and BC in the rectangle ABCD are 3 and 5 cm respectively. If AA' BB' CC' DD' x= = = = , express the area of the rhomboid A’B’C’D’ as a function of x. Exercise 25: The number value of the polynomial 34 x 3 π express the volume of a sphere with radius x, and the number value of the polynomial 2 4 xπ express the area of a sphere with radius x. a) Is there any sphere whose volume (expressed in m3 ) is the same as its area (expressed in m2 )? If your answer is affirmative, find the radius of that sphere. b) Find the relation between the area of a sphere and the area of a maximum circle in that sphere. c) Use a polynomial of x to express the volume of the cylinder circumscribed in a sphere with radius x. Find the relation between this polynomial and the one which express the volume of the sphere.