Deflection in beams

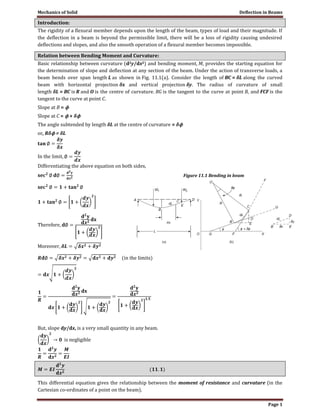
Mechanics of Solid Deflection in Beams
Page 1
Introduction:
The rigidity of a flexural member depends upon the length of the beam, types of load and their magnitude. If
the deflection in a beam is beyond the permissible limit, there will be a loss of rigidity causing undesired
deflections and slopes, and also the smooth operation of a flexural member becomes impossible.
Relation between Bending Moment and Curvature:
Basic relationship between curvature (d2y/dx2) and bending moment, M, provides the starting equation for
the determination of slope and deflection at any section of the beam. Under the action of transverse loads, a
beam bends over span length L as shown in Fig. 11.1(a). Consider the length of BC = δL along the curved
beam with horizontal projection δx and vertical projection δy. The radius of curvature of small
length δL = BC is R and O is the centre of curvature. BG is the tangent to the curve at point B, and FCF is the
tangent to the curve at point C.
Slope at B = ϕ
Slope at C = ϕ + δϕ
The angle subtended by length δL at the centre of curvature = δϕ
or, Rδϕ = δL
Differentiating the above equation on both sides,
But, slope dy/dx, is a very small quantity in any beam.
This differential equation gives the relationship between the moment of resistance and curvature (in the
Cartesian co-ordinates of a point on the beam).
Figure 11.1 Bending in beam

Page 2
Sign Conventions:
1.On the left side of a section, upward shear force is positive or the shear force tending to rotate the body in
clockwise direction is positive.
2. On the left side of the section, clockwise bending moment is positive, the bending moment which produces
concavity upwards is a positive bending moment.
3. In x–y Cartesian co-ordinate system, x is positive towards right and y is positive in upward direction.
Figure 11.2 shows a beam AB in bent shape showing concavity upwards, the bending moment from A to B is
positive. The deflection at A, yA, and the deflection at B, yB, are positive, while the deflection at C, yC, is
negative (below x axis). Similarly the slope at E, iE, is positive, while the slope at D, iD, is negative.
Figure 11.2 Slopes and deflections in beam
Simply Supported Beam with a Central Point Load:
A beam of length L, hinged at end A and roller supported at end B, carries a central point load W at centre C.
Flexure curve of the beam is ACB, Fig 11.3. The slope at A is –i′A, the slope at B is –i′B, and the slope at C is zero
due to symmetrical loading. Similarly, the deflection at ends A and B is zero but the deflection at centre C is yC,
the maximum. Due to symmetry, reactions at A and B will be equal, that is,
Consider a section YY at a distance of x from end A.
Then, bending moment,
Using the equation 11.1 of bending moment and curvature,
Integrating Eq. (11.2), we get
where C1 is a constant of integration.
At the centre, x = L/2, dy/dx = 0, and the slope is zero
From the above equation, we cannot find the slope at B because we have made equation of bending moment
only in portion AC of the beam.
Integrating Eq. (11.3) again,
Figure 11.3

Page 3
where C2 is another constant of integration.
The deflection y = 0 at x = 0, at end A,
0 = 0 − 0 + C2
Hence, constant, C2 = 0
Finally,
Deflection at C, x = L/2, by substituting the value in Eq. (11.4),
A Beam Carrying Udl with Simply Supported Ends:
A beam AB, simply supported at ends over a span L, carries a udl (uniformly distributed load) of
intensity w per unit length. Total load on beam = wL, due to symmetrical loading about the centre of the
beam.
Reactions, RA = RB = wL/2, Fig. 11.4.
Considering a section at a distance of x from end A, then the bending moment at this section is
In this case, Eq. (11.5) is sufficient to determine the slope and deflection at any section of the beam as there is
only one portion, AB.
Integrating Eq. (11.5),
Slope dy/dx = 0, at x = L/2, by substituting this value,
Integrating Eq. (11.6),
where C2 is another constant of integration at end A, x = 0, deflection, y = 0.
So, 0 = 0 − 0 + C2
or constant, C2 = 0.
Finally,
Figure 11.4

Page 4
This shows that expression (11.7) is valid from one end to other end of beam.
At the centre, x = L/2, the deflection is maximum, therefore,
Slope is maximum at ends, when x = 0, from Eq. (11.6),
At end B, x = L, by substituting this value in Eq. (11.6),
A Cantilever with the Point Load at Free End:
Figure11.5 shows a cantilever AB of length L, free at end A and fixed at end B, carrying a point load W at A.
Considering a section YY at a distance of x from A.
Bending moment, Mx = −Wx (bending moment producing convexity upwards)
Integrating Eq. (11.8)
at fixed end x = L, slope dy/dx, so
Integrating Eq. (11.9), we get
at x = L, fixed end, deflection, y = 0
Finally,
Figure 11.5 Cantilever with point load at free end

Page 5
Note that both slope and deflection are maximum at free end A, where x = 0.
;
A Cantilever with a UDL:
A cantilever AB of length L, free at end A and fixed at end B carrying a uniformly distributed load of
intensity w per unit length, is shown in Fig. 11.6. The cantilever bends as shown, point A is shifted to A′. Slope
and deflection are maximum at free ends.
Total load on the cantilever = wL′
Reaction at B, RB = wL′
Fixing moment at B, MB = wL2/2 as shown.
Consider a section YY at a distance x from end A.
Bending moment, Mx = Mx = –wx2/2 (bending moment tending to produce convexity is negative)
Integrating
At end B, fixed end, slope dy/dx = 0
Integrating again, we set
Deflection y = 0, at fixed end B, where x = L
Finally,
At end A, x = 0.
Slope and deflection at any section of the cantilever from A to B can be determined by using Eqs
(11.11) and (11.12).
Example: A steel cantilever of I section with Ixx = 1,200 × 104 mm4, and length 4 m carries a udl of
intensity w kN/m. If the maximum deflection in cantilever is not to exceed 1 mm, E = 208 GPa. Determine the
value of w.
Solution: E = 208 × 106 kN/m2 ; I = 1,200 × 10−8 m4 ; EI = 2,496 kN m2
Say, rate of loading = w kN/m
Length, L = 4 m
Figure 11.6 A cantilever with a udl

Page 6
Maximum deflection, ymax = wL4/8EI = 1 mm = 1 × 10−3 m
or
Macaulay’s Method:
This is the most versatile technique to determine slope and deflection at any section of a beam/cantilever
carrying any type of loading or a combination of loading such as point loads, udl, moment or a variable load.
In this method following steps are taken:
1. Determine the reactions at supports using the equations of equilibrium.
2. Select any one end as origin and go to the last portion of the beam (separated by loads) and take x from
origin to a section YY in the last portion.
3. Make the equation of bending moment for the section under consideration.
As example shown in fig. Last portion is DE, BM at section YY
4. Integrate this equation two times with constants of integration, C1 and C2.
5. Use boundary conditions of slope and deflection at ends and by carefully using the terms of the equation
which are valid for that end, determine constants C1 and C2.
6.These constants will be valid for all portions of the beam.
7. Once the equations for deflection and slope with known constants C1 and C2 are made, then slope and
deflection can be calculated at any section of the beam.
Example: A beam AB, 10 m long, carries point loads of 6 and 3 kN at C and D as shown in Fig.. Determine
support reactions, deflection at C and D, and slope at ends A and B, if EI is the flexural rigidity of the beam.
Solution:
Reactions
Taking moments about A,
6 × 4 + 7 × 3 = 10RB
Reaction, RB = (24 + 21)/10 = 4.5 kN
Total load on beam = 6 + 3 = 9 kN
Reaction at A, RA = 9 − RB = 9 − 4.5 = 4.5 kN.
There are three portions, that is, AC, CD and DB in the beam and if A is the origin then DB is the last portion.
Consider a section at a distance x from A in the portion DB,
Bending moment, Mx = 4.5x − 6(x − 4) − 3(x − 7)
or
Integrating
Since the beam is not symmetrically loaded about its centre, so we do not know where slope is zero.
By integrating

Page 7
Boundary conditions x = 0 at end A, deflection y = 0
Moreover in portion AC, only the first term is valid and the other two terms of equation are not valid.
So, 0 = 0.75 × 0, neglected term – neglected term + C1 × 0 + C2
or, constant C2 = 0
At end B, x = 10 m, deflection y = 0, by substituting the value
0 = 0.75 × 103 – 63 – 0.5 × 33 + 10C1
0 = 750 – 216 –13.5 + 10C1
Constant, C1 = –52.05
Finally, the equations are
EIy = 0.75x3 − (x − 4)3 − 0.5(x − 7)3 − 52.05x (11.16)
Slope at end A, x = 0
EIiA = 2.25 × 0 – neglected terms –52.05
or
at end B, x = 10, so all the terms are valid
Deflection
At point C, x = 4 m, hence, the third term in the equation is invalid.
EIyC = 0.75 × 43 − (4 − 4)3 − neglected term − 52.05 × 4
EIyC = 48 − 0 − 208.2 = −160.2
At point D, x = 7 m, and all the terms in the equation for deflection are valid.
EIyD = 0.75 × 73 − (7 − 4)3 − 0.5(7 − 7)3 − 52.05 × 7
257.25 − 27 − 0 − 364.35= −134 1
Example: A beam ABCD, 6 m long hinged at end A and roller supported at end D, is subjected to CCW moment
of 10 kN m at point B and a point load of 10 kN at point C as shown in Fig. Determine the deflection under
load of 10 kN and slope at point B, by taking EI as flexural rigidity of the beam.

Page 8
Solution:
Taking moments at A,
10 + RD × 6 = 10 × 4
RD = 5kN ↑
Total load on the beam = 10 kN
Reaction at A, RA = 10 – 5 = 5kN ↑
There are three portions: AB, BC and CD in beam, with A as the origin portion and CD is the last. Take a
section YY in portion CD at a distance x from A as shown in the figure.
The equation of bending moment is
Note that 10 kN m is a moment and we cannot take moment of moment, but 10 kN m is applied at B at a
distance of (x – 2) m from section YY. Moreover (x – 2)°= 1, so only to locate the position of moment, the term
(x – 2)° is taken, that is, (x – 2) raised to power zero, this term locates the position of moment applied at B.
Integrating we get
where C1 is a constant of integration, we do not know where the slope is zero as the beam is not
symmetrically loaded.
Also by integrating
where C2 is another constant of integration. y = 0 at end A, x = 0, only first two terms in between are valid in
portion AB, so
or, constant, C2 = 0.
y = 0 at end D, where x = 6 m, all the terms in the equation are valid, so
Finally, the equations for slope and deflection are
The slope at B and x = 2 m, the third term is not valid
Deflection at C, x = 4 m, all the terms in equation for deflection are valid for x = 4 m

Page 9
Example: A beam ABCD, 7 m long hinged at A and roller supported at D carries 7 kN load at B and 4 kN/m udl
over BC = 3 m. If EI = 14,000 kN m2 for the beam, determine the slope at A and deflection at point C.
Solution:
Reactions
Total udl on beam = 4 × 3 = 12 kN
CG of this load lies at 2 + 1.5 = 3.5 m from end A
Taking moments about A,
7 × 2 + 4 × 3(3.5) = 7 RD
RD = 8 kN ; RA = 11 kN
Last portion of the beam is CD. A section YY at a distance x from end A in the portion CD of the beam is taken
to make the equation of bending moment valid for all the three portions. The udl is extended to section YY on
both sides (upward and downward), so that its net effect becomes zero.
Bending moment at section YY
where w is the rate of loading.
Note that the first term is valid for portion AB, the first three terms are valid for portion BC, and all the four
terms are valid for portion CD of the beam.
Substituting the value of w = 4 kN/m,
Integrating
Integrating
At end A, x = 0, y = 0 (in portion AB, x = 0, their three terms are invalid)
Constant, C2 = 0.
At the end D, x = 7 m, y = 0, and all the terms in equation are valid.
Constant, C1 = –54.5
The equations will become
Slope at A, x = 0

Page 10
Deflection at C, x = 5 m, and all the terms are valid
Eccentric Load on a Beam:
A beam AB of length L, hinged at end A and roller supported at end B, carries a load W at point C such that,
AC = a; CB = b; a + b = L
As shown in fig. a<b. In this case, we do not know where the slope is zero but certainly we know that the
deflection at ends A and B is zero.
Reactions
Taking moments about A, Wa = RBL
Reaction, RB = Wa/L
By taking the origin at A and x is positive towards right at section YY at a distance x from A in the portion CB.
Integrating two times, we obtain
At end A, y = 0, x = 0
EI × 0 = 0 − neglected terms + 0 × C1 + C2
Constant, C2 = 0
At end B, y = 0, x = L
The expression for slope and deflection will be
Deflection under the load x = a

Page 11
If a = b = L/2 (for central load)
Example : A beam ABC, 8 m long carries an eccentric load at B, such that AB = 3 m, BC= 5 m. If EI = 5,000 kN
m2, determine (1) slope at ends A and C, and (2) maximum deflection.
Solution:
Reactions
Taking moment about A, 8 × 3 = 8 Rc
Reaction, Rc = 3 kN
Reaction, RA = 8 – 3 = 5 kN
Taking a section in portion BC,
Mx = 5x – 8(x – 3)
Integrating two times, we obtain
where C1 and C2 are constants of integration. At x = 0, y = 0,
EI × 0 = –omitted term + 0 × C1 + C2
Constant, C2 = 0
At x = 8 m, end C, deflection y = 0
Equation for slope will become
At A, x = 0
At C, x = 8 m
ymax occurs in the beam where slope dy/dx is zero. Let us find location of ymax’
x = 3.718 m
Substituting the value of x in equation of deflection,

Page 12
Impact Loading of a Beam:
If a load falls from a height onto a beam, instantaneous deflection is produced in the beam, causing
instantaneous stress of high level in the beam and the beam starts vibrating, but ultimately the vibrations die
down as the amplitude of vibration goes on decreasing due to air damping. Consider a beam AB of
length L and flexural rigidity EI as shown in Fig. 11.16. A load W falls from a height h on the beam and the
deflection under the load δi is produced in the beam.
Loss of potential energy of the falling weight = W (h + δi)
Strain energy absorbed by the beam = 1/2Pδi,
where P is the equivalent gradually applied load on the beam which when applied gradually produces
deflection δi.
Say the load falls at the centre of the beam,
then
where K = stiffness constant of beam =48EI/L3
If W and h are given then δi can be calculated. The maximum instantaneous stress developed due to δi can also
be calculated. Note that once the vibrations die down. δi will approach
Example: An ISMB 150 rolled steel section is held as a cantilever of length 2 m. A weight of 200 N is dropped
at the free end of the cantilever producing an instantaneous stress of 90 N/mm2. Calculate the height from
which the weight was dropped and the maximum instantaneous deflection in the cantilever. I = 726.4 × 10–
8 m4, E = 200 GPa
Solution
Length of the beam = 2 m
Say, equivalent load = P kN
Mmax, maximum bending moment = 2P kN m
σmax, maximum stress developed = 90 MPa = 90 × 106 N/m2 = 90 × 103 kN/m2
EI = 726.4 × 10-8 × 200 × 106 = 1,452.8 kN m2
Depth = 0.075 m (Note that 150 stands for 150 mm as depth of beam)
Instantaneous deflection,
Maximum instantaneous deflection, δi = 0.008 m
W = 200 N = 0.2 kN
h = 0.087108 – 0.008 = 0.07917 m = 79.17 mm
Figure 11.16

Page 13
Propped Cantilevers:
A cantilever fixed at one end and simply supported at other end is known as propped cantilever. The propped
cantilevers are of two types:
1. A known force P is applied at the free end in a direction opposite to the direction of applied load W as
shown in Fig. 11.17.
Bending moment equation is
Taking the boundary conditions at end B, that is, x = L, y = 0, dy/dx = 0, constants of integration are found
out. Hence, the slope and deflection at any section of the cantilever can be determined.
2.Free-end of the loaded cantilever is simply supported so that the deflection at free end is zero, by knowing
the boundary conditions at A and B, constants C1 and C2and reaction at A, that is, RA are determined as
shown in Fig. 11.18.
Equation of bending moment for a section YY in portion CB,
Mx = RAx – W(x – a)
Figure 11.17 Propped cantilevers Figure 11.18 Propped cantilevers
Integrating
There are three boundary conditions, that is, x = 0, y = 0, x = L, y = 0, dy/dx = 0, thus one can
determine RA, C1 and C2, (3 unknowns.)
Example: Cantilever AB, 5 m long, is simply supported at A and fixed at B. If it carries a udl of 6 kN/m
over CB = 3 m, EI of cantilever is 3,600 kN m2. Determine the reaction at Aand slope at A and also find out the
deflection at C (Fig. 11.20)?
Solution:
Say reaction at A is RA. Taking a section at distance x from A, in the portion CB,
Bending moment,
where w is rate of loading
or,
Integrating we get
where C1 is a constant of integration; dy/dx = 0 at x = 5, by substituting this value, we obtain
Figure 11.20

Page 14
So,
Also by integrating
At A, x = 0, y = 0
C2 = 0
Finally,
Moreover, y = 0 at end B, x = 5 m, by substituting this value,
By substituting the value of RA, equations of slope and deflection are
At A, x = 0
Deflection at C, x = 2 m
Stepped Beam:
Consider a beam AB of length L, with moment of inertia I1 for AC and I2 for CB portions of the beam, which is
subjected to a central point load W. Let us determine the deflection at the centre of the beam.
Reactions are RA = RB = W/2 (as shown).
Taking portion CB, the equation of bending moment becomes
The above equation shows the variable moment of inertia. The equation is modified because the section of the
beam is not uniform.
Integrating two times, we obtain,
at x = 0, y = 0
0 = 0 – omitted term + 0 + C2
Constant,C2 = 0
Figure 11.23

Page 15
at x = L, y = 0, other end B
By substituting the value of RA = W/2,
Therefore,
At the centre x = L/2,
Say I1 = I2 = I
Let us take I1 = 2I2
Slope and Deflection by Area Moment Method:
Slope and deflection at any section of a beam can be obtained by (a) area of bending moment diagram, and (b)
first moment of area of BM diagram.
Equations of curvature and moment are
multiplying both order by dx
Mdx is the area of BM diagram over a small length dx.
Integrating both sides
area of BM diagram between sections Y2Y2 and Y1Y1
or between distances x2 and x1
or i2 − i1 = area of BM diagram between Y2Y1.

Page 16
Multiply by xdx on both the sides, and then integrating, we get
a = area of diagram abcdef.
= distance of CG of this diagram from A
Example: A simply supported beam of span length L carries a udl of intensity w throughout its length.
Determine the slope at A and deflection at C by moment area method.
Solution: BM diagram is parabolic for this case with maximum bending moment at centre = + wL2/8
Area of the bending moment diagram from A to C that is, area AC′C
Because of symmetrical loading, ic = 0, and slope at centre is zero.
Now area,
CG of area AC’C lies at a distance of (5/8 × L/2) from A.
Now,
But xc = L/2, ic = 0
Therefore, xA = 0, yA = 0
Finally,
Conjugate Beam Method:
In this method, bending moment diagram for beam due to transverse loads on it is considered as loading
diagram (but in term of variation of bending moment). Taking this bending moment diagram as loading
diagram on the beam, reactions are calculated at supports (in term of bending moment). Thus,

Page 17
Figure 11.27 Figure 11.28
Deflection
Moment at any section due to variable bending moment can be determined, say M′c is moment at C due to
bending moment diagram AC′ D′B, and M′D is the moment at D due to variable moment AC′ D′B, then,
A simple example will help in understanding the concepts of conjugate beam method. A beam AB of length L is
simply supported at ends, which carries a concentrated load at the centre as shown in Fig. 11.28. EI is the
flexural rigidity of the beam.
Figure 11.28(b) shows the bending moment diagram of the beam with maximum bending moment, WL/4 at
the centre supported over length L, at A and B.
Reactions at A and B are given as
At the point C, moment
Slope and Deflection of Stepped Beams:
For stepped cantilevers/beams, conjugate beam method is very conveniently applied. In such cases, bending
moment diagram is plotted for the beam/cantilever with bending moment diagram as a load diagram and the
reactions at ends are obtained. The ratio of reaction/EI gives slope at the end, ratio of moment (of bending
moment) divided by EI gives deflection at any section. In case of cantilever, maximum slope and deflection
occur at free end, while both slope and deflection are zero at fixed end. Therefore in conjugate beam method,
cantilever free end becomes the fixed one and the fixed end becomes the free one, so that the reaction and
moment can be obtained at this fixed end (which is initially free end) of the cantilever.
Example: A beam of length L carries a central load W as shown in Fig. 11.32(a). Moment of inertia for quarter
length from ends is I1 and for the middle half length moment of inertia is I2, such that I2 = 2I1, now draw the
conjugate beam diagram.
Solution:

Page 18
If E is Young’s modulus of the material, diagram AE′B is the bending moment diagram such that EE’ = WL/4
Conjugate beam diagram gives,
Beam is symmetrically loaded, therefore reactions, RA′ = RB′.
RA′ = area of conjugate beam diagram up to centre.
iE = slope at centre is zero
iE – iA = area of conjugate beam diagram up to centre.
Moment at centre, M′E
Figure 11.32

Deflection in beams

Recomendados

Recomendados

Más contenido relacionado

La actualidad más candente

La actualidad más candente (20)

Similar a Deflection in beams

Similar a Deflection in beams (20)

Más de Yatin Singh

Más de Yatin Singh (20)

Último

Último (20)

Deflection in beams