gsddgfdgdfgd

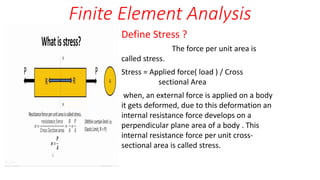
1. Finite Element Analysis
Define Stress ?
The force per unit area is
called stress.
Stress = Applied force( load ) / Cross
sectional Area
when, an external force is applied on a body
it gets deformed, due to this deformation an
internal resistance force develops on a
perpendicular plane area of a body . This
internal resistance force per unit cross-
sectional area is called stress.

2. What is strain ?
The strain is defined as the ratio of change
in dimension onto the original dimension .
Strain is the measure of how much
distortion has been fallen on a body compare3d to
its initial shape due to action of force.
Types of Strain:
Longitudinal strain = Ratio of change in length
to the original length .
Shearing Strain = Ratio of change in angle to
which it is turned to its distance from the fixed
layer.
Volumetric Strain = Ratio of change in volume to
original volume.

3. Young’s modulus :
The Young’s modulus (E), is a property of the
material that tells us how easily it can be stretch
and deform.
It is defined as the ratio of tensile stress to
tensile strain.

4. Rigidity modulus:
Shear modulus is also known as modulus of
rigidity.
The ratio of shear stress to shear strain,
determines the modulus of rigidity.
Within the elastic limit, it is the ratio of shear
stress to shear strain
It is associated with the change in the shape
of a body.
It exists in solids only.
It describes an object’s tendency to shear.

5. Bulk Modulus ?
Bulk modulus is a numerical constant that
describes a solid’s or fluid’s elastic properties
when it is in pressure on all sides.
When the pressure is applied to a material, it
reduces its volume, which then returns to its
original volume when the pressure is removed.

6. Stiffness :
Stiffness is the extent to which an object resists
deformation in response to an applied force.
where,
where,

7. Linear & Non-Linearity :
A linear analysis uses only linear elastic
materials and small displacements.
A non-linear analysis uses large
displacements and elastoplastic materials, the
superposition effect cannot be used.

8. Natural frequency :
A natural frequency is a frequency at which a body will
vibrate if excited by an external force.
when, an object vibrates at a frequency equivalent to
its natural frequency, the vibration of the amplitude
increases significantly which could lead to irreparable
damage, therefore, it is important to know the natural
frequency.

9. Resonant frequency:
The natural frequency of an object, where it
tends to vibrate at a higher amplitude, is also
known as the resonant frequency.
For Example : If a collective oscillation force
from the automobiles caused a bridge to vibrate
at its frequency, we may feel it shake.

10. Damping:
Damping in Physics, restraining of vibratory
motion, such as mechanical oscillations, noise, and
alternating electric currents, by dissipation of energy.

11. Properties of materials:
Hardness – Resistance to scratching, cutting or abrasion.
Toughness – Resistance to fracturing and this quality depends on the maximum
energy that can be absorbed before fracturing.
Brittleness – They are liable to break easily, they are hard and can’t be hammered.
Ductility – They can easily be hammered or stretched into thin wires without
breakage.
Malleability – Capability of being shaped or extended by hammering, forging, etc…
Strength – The ability of a material to resist mechanical forces when in use. A
materials strength in a given application depends on many factors, including its
resistance to deformation and cracking, and it often depends on the shape of the
member being designed.

12. Finding Natural frequency:
When an object vibrates at a frequency equivalent to its
natural frequency, the vibration of the amplitude increases
significantly, which could lead to irreparable damage,
therefore, it is important to know the natural frequency.

13. Finite Element Method:
Finite Element Method is a numerical technique for
solving boundary value problems in which a large domain is
divided into smaller sections or elements.
The solution is determined by assuming certain
polynomials.
The small elements are called finite elements and the
polynomials are called shape functions.

14. Advantages of FEM:
Since the properties of each element are evaluated
separately, different material properties can be
incorporated for each element.
There is no restriction in the shape of the medium.
Any type of boundary condition can be adopted.

15. Various co-ordinates in the FEM:
• Local coordinates
• Natural coordinates
• Simple natural coordinates
• Area coordinator
• Triangular coordinates
• Generalized coordinates

16. Basic steps of FEM:
• Discretization of the structure.
• Selection of sustainable displacement function.
• Finding the element properties.
• Assembling the element properties.
• Applying the boundary conditions
• Solving the system of equations
• Computing additional results

17. Different types of elements in FEA:
It is the process of subdividing the given body into a
number of elements which results in a system of equivalent
finite elements.
• 1D
• 2D
• 3D
Discretization:

18. Why should one use Finite elements?
The use of finite elements allows the modelling of
multiple material types, testing of complex geometry, and the
ability to capture local effects acting on a small area of the
design.
In practice, engineers can use finite element modelling
software on a huge variety of tasks.

19. Node or Joint:
Each kind of finite elements has a specific structural
shape and is interconnected with the adjacent element
by nodal point or nodes.
At nodes, degrees of freedom are located. The forces
will act only at nodes at any others place in the
element.

20. Types of Boundary Conditions:
• Dirichlet Boundary Condition
• Neumann Boundary Condition
• Robin Boundary Condition
• Mixed Boundary Condition
• Cauchy Boundary Condition

21. Three phases of FEM:
• Classification of the problem
• Discretization
• Modelling

22. DOF:
• The degree of freedom of a mechanical system is
the number of independent parameters that
define its configuration or state.
• It is important in the analysis of systems of
bodies in mechanical engineering, structural
engineering, aerospace engineering, robotics and
other fields.

23. Types of FEA:
• Structural Analysis
• Thermal Analysis
• Fluid Analysis
• Heat Transfer Analysis
• Electromagnetic Analysis
• Buckling Analysis
• Electrical Analysis
• Multi Physics Analysis ( coupled field ).